Prudential fiscal stimulus

ZEW Public Finance Conference, 4 May 2023

Alfred Duncan

University of Kent

Charles Nolan

University of Glasgow

This project is supported by research funding from UKRI Grant Ref: ES/V015559/1

1 / 33

The conventional view (the Greenspan put)

Stimulus policies increase moral hazard.

Anticipating to be rescued in downturns, firms might take more risk today.

2 / 33

The theorem (Arnott-Greenwald-Stiglitz)

Policymakers can reduce moral hazard, and increase efficiency,
by taxing or regulating the complements of moral hazard and/or
subsidising the substitutes for moral hazard.

Doesn't align with concerns about the Greenspan put.

We ask, when does fiscal stimulus reduce the moral hazard
problems that concern prudential policymakers?

3 / 33

Our contribution

We show that countercyclical wage subsidies can improve welfare, by reducing the cost of moral hazard frictions in firm financing.
Result holds even in the absence of aggregate demand externalities.
We characterise the optimal wage subsidies.
We estimate that simple rule implementations can generate large welfare gains.

Figure: Countries with new or existing wage subsidy schemes during the Covid-19 pandemic (Sources: ILO, IMF, authors’ calculations)

4 / 33

Intuition behind our result

Absent intervention

5 / 33

Intuition behind our result

Introduction of the wage subsidy

6 / 33

Intuition behind our result

Firm's risk allocation response

7 / 33

Intuition behind our result

8 / 33

Intuition behind our result

Labour supply is a complement to firms' inside wealth.

Anticipating wage subsidies in recessions, firms will be more prudent in expansions.

The ex-post wage subsidy replicates an ex-ante macroprudential intervention.

9 / 33

Macropru

di Tella (2017); Duncan and Nolan (2022).
Farhi and Werning (2016); Schmitt-Grohe and Uribe (2012);

Information economics

Arnott and Stiglitz (1991)

10 / 33

The macroprudential externality11 / 33

The macroprudential externality

Three ingredients

Anonymous unrestricted trade in aggregate-state contingent securities.
Agency costs restricting trade in idiosyncratic-state contingent securities.
Risk aversion over individual specific states.

Jump to results

12 / 33

The entrepreneur

combines their own wealth with borrowed wealth and labour.

Contracts are endogenously incomplete. Entrepreneurs can hide income from external creditors.

External creditors can audit the firm and uncover hidden income, but these audits are noisy.

(Duncan and Nolan, 2019)

13 / 33

The entrepreneur's intratemporal problem

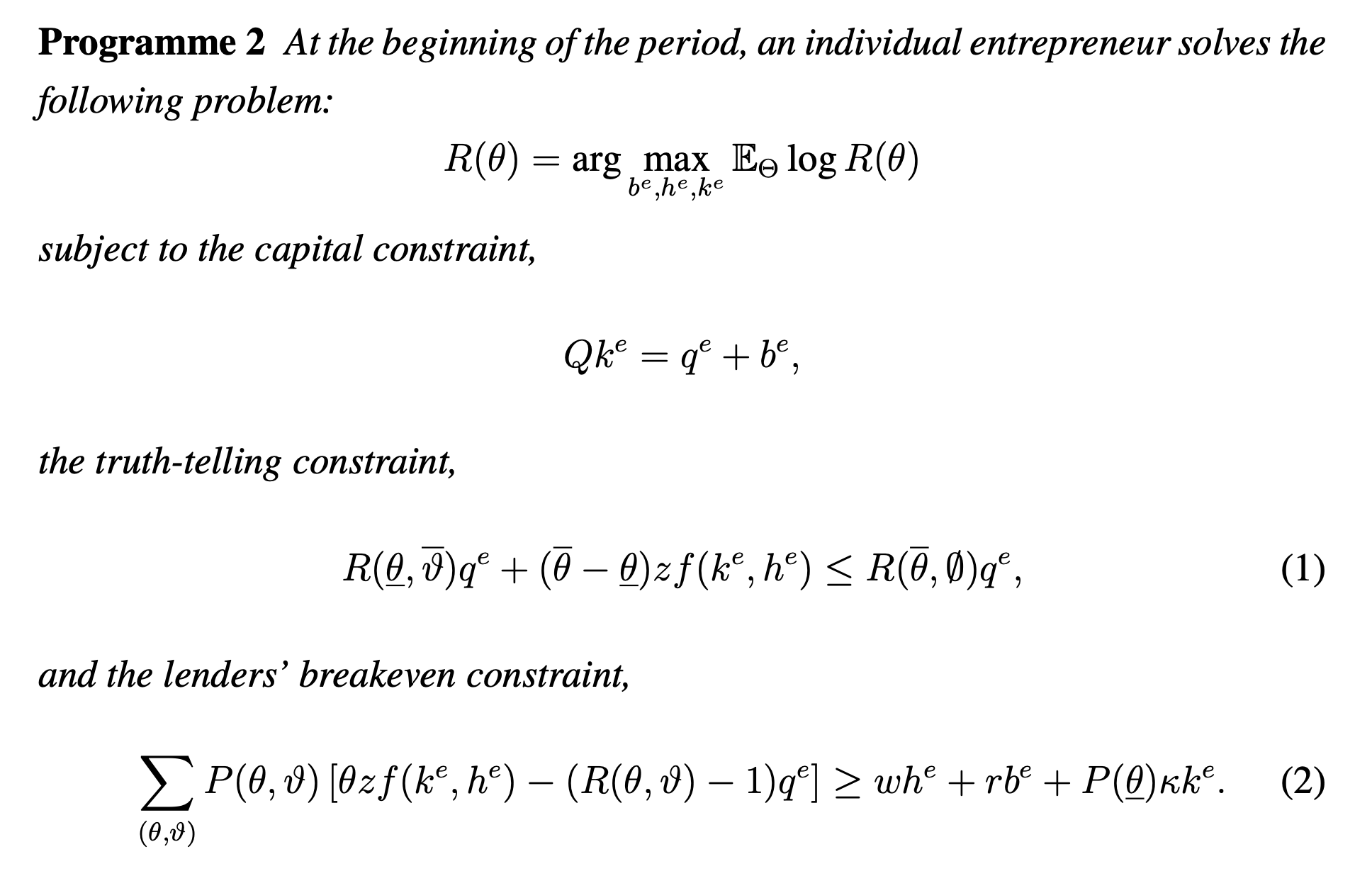
Extension
We present an extension using an alternative financial friction applied to a financial intermediary sector. The main results of the paper go through.

14 / 33

The entrepreneur's intertemporal problem

\int_{s^{'} \in S} p (s^{'}) x^{e} (s^{'}) d s + x^{e} (s^{'})

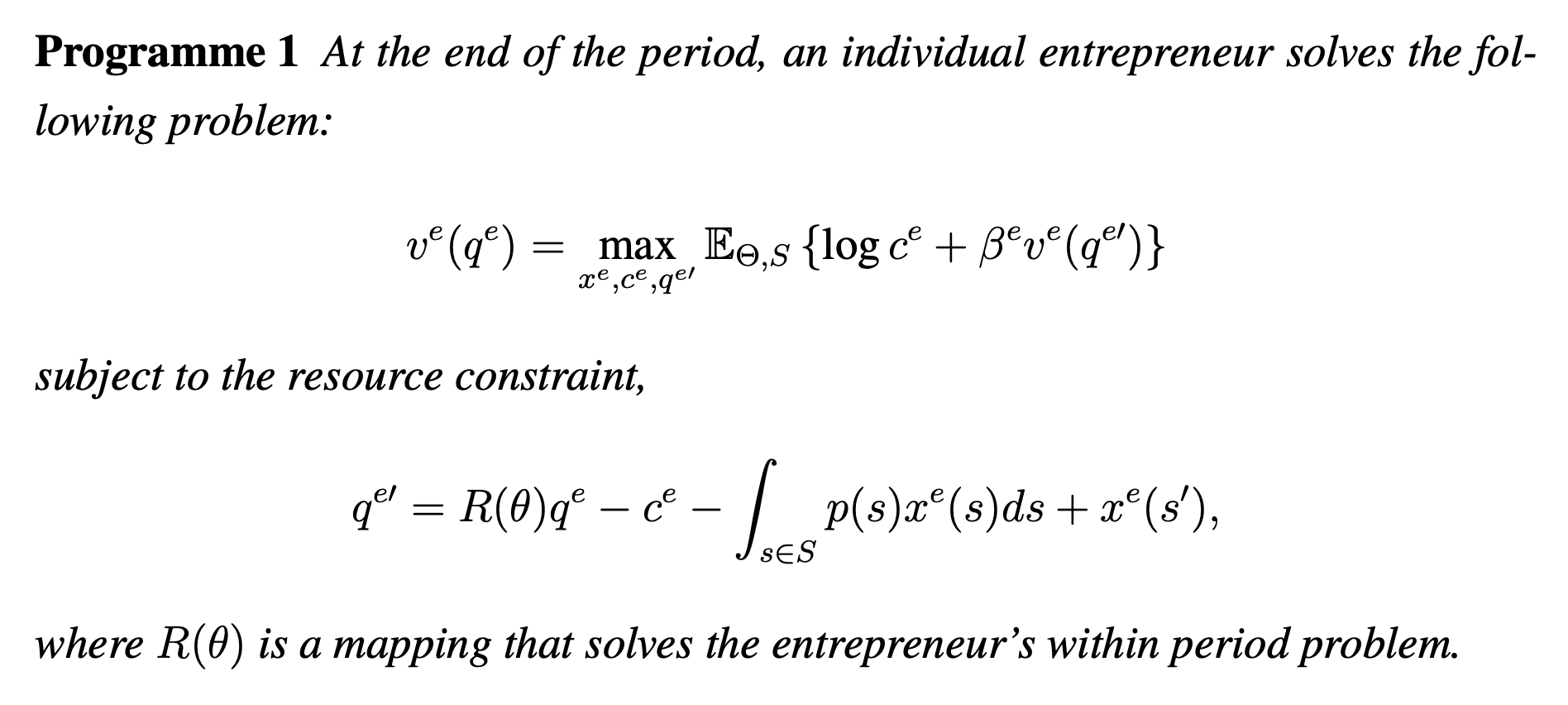
captures trade in aggregate state contingent securities.

Markets for aggregate risks are complete.

15 / 33

The household's problem

v (q) = max_{x, c, h, q^{'}} E {u (c, h) + β v (q^{'})}

subject to

$q^{'} = (1 + r) q + w h - c - \int_{s^{'} \in S} p (s^{'}) x (s^{'}) d s + x (s^{'})$

\int_{s^{'} \in S} p (s^{'}) x (s^{'}) d s + x (s^{'})

captures trade in aggregate state contingent securities.

Markets for aggregate risks are complete.

16 / 33

Factor markets

$l = \frac{E_{Θ} f (θ, k, h)}{(1 + r) q^{e}}$

$\frac{E_{Θ} [R (θ, s)]}{1 + r} = 1 + l τ$

$w = E_{Θ} f_{h} (θ, k, h) (1 - τ)$

Leverage, l

17 / 33

Factor markets

$l = \frac{E_{Θ} f (θ, k, h)}{(1 + r) q^{e}}$

$\frac{E_{Θ} [R (θ, s)]}{1 + r} = 1 + l τ$

$w = E_{Θ} f_{h} (θ, k, h) (1 - τ)$

The equity risk premium,

$ρ := \frac{E_{Θ} [R (θ, s)]}{1 + r}$

18 / 33

Factor markets

$l = \frac{E_{Θ} f (θ, k, h)}{(1 + r) q^{e}}$

$\frac{E_{Θ} [R (θ, s)]}{1 + r} = 1 + l τ$

$w = E_{Θ} f_{h} (θ, k, h) (1 - τ)$

Wages, w

19 / 33

The competitive allocation of aggregate risk

$\frac{β^{e} {E_{Θ}^{'} u^{e}}^{'} ({c^{e}}^{'} (θ^{'}))}{{u^{e}}^{'} (c^{e})} = \frac{β u^{'} (c^{'}, h^{'})}{u^{'} (c, h)}$

20 / 33

Optimal macroprudential policy

$(1 + ω) \frac{β^{e} {E_{Θ}^{'} u^{e}}^{'} ({c^{e}}^{'} (θ^{'}))}{{u^{e}}^{'} (c^{e})} = \frac{β u^{'} (c^{'}, h^{'})}{u^{'} (c, h)}$

Under optimal policy $\frac{\partial ω}{\partial l}, \frac{\partial ω}{\partial σ} > 0.$

Optimal macroprudential policy leans against

fluctuations in leverage, and
entrepreneurs' exposure to risk shocks.

21 / 33

The macroprudential externality

Cyclical risk is a complement to downturn moral hazard.
Entrepreneurs accept too much cyclical risk,
amplifying the cost of moral hazard in downturns,
Arnott-Stiglitz: Regulate cyclical risk (macroprudential)

22 / 33

Optimal wage subsidy policy23 / 33

Optimal wage subsidy policy - example with log utility

Proposition

Let $u (c, h) = \log c - \frac{h^{1 + ψ}}{1 + ψ}, u^{e} (c^{e}) = \log c^{e} .$

a. Optimal wage subsidy: $ς^{*} = \frac{τ}{1 - τ} (1 - (\frac{l_{0} ρ - l ρ_{0}}{ρ - 1}) \frac{(1 - β^{e})}{l_{0} - (1 - β^{e}) ρ_{0}}) .$

b. Output is completely stabilised in response to uncertainty shocks, and is proportional to total factor productivity.

24 / 33

Optimal wage subsidy policy - example with log utility

Under the competitive equilibrium in the absence of wage subsidy policy, real output follows

$y = z {(\frac{α l (1 - τ)}{l - (1 - β^{e}) ρ})}^{\frac{α}{1 + ψ}} .$

Under the optimal wage subsidy, real output follows

$y = z {(\frac{α l_{0}}{l_{0} - (1 - β^{e}) ρ_{0}})}^{\frac{α}{1 + ψ}}$

Real output is proportional to total factor productivity in both regimes, but only responds to fluctuations in leverage and risk under the competitive equilibrium.

Under the optimal wage subsidy regime, output does not respond to uncertainty shocks.

25 / 33

How the intervention works

Benefit

Wage subsidies

complement firms' wealth,
encourage precaution during expansions, and
decreases financial frictions in downturns.
First order welfare gain.

Cost

Wage subsidies

introduces a distortion between labour supply and demand,
Second order welfare cost.

26 / 33

Quantitative exercise27 / 33

Exercise

We estimate the model on US business cycle data, with no macroprudential or wage subsidy policy.
We add a wage subsidy, via a simple rule, and find the optimal simple rule and the associated welfare gain.

28 / 33

Wage subsidy simple rule

We propose the following simple rule:

$ς = - ϕ_{ς} (y - y_{0})$

where $ς$ is the wage subsidy (tax if negative), and $y_{0}$ is deterministic steady state output.

29 / 33

Expected welfare effects of wage subsidy simple rules

Welfare gain is expressed as a share of business cycle welfare losses.
Shaded area indicates 90% credible interval.

30 / 33

Persistence of TFP shocks

Welfare gain is expressed as a share of business cycle welfare losses.

31 / 33

Extensions

Commitment vs discretion

Optimal wage subsidy policy generates an ex post distortion, that reduces efficiency. The benefit of the policy derives from changes in agents' actions ex ante.

In the flexible price model, a policymaker optimising under discretion does not use wage subsidy policies.

Sticky prices

(Dixit's critique) If the margin that the policy is acting on is distorted,
then the Arnott-Stiglitz logic doesn't necessarily hold.

New Keynesian markups act on same margin as policy. We verify that New Keynesian frictions do not overturn the result.

In fact, in the NK extension, the commitment problem is less severe, as there is an ex post incentive to stimulate the economy.

32 / 33

Summary

We present a model where moral hazard generates a macroprudential externality.

In lieu of aggregate demand externalities, there is still a role for fiscal stimulus.

If the stimulus programme complements inside wealth, like a labour subsidy, then it will

encourage firms' prudence during the preceding expansion, and
reduce the costs of the moral hazard friction,
increasing welfare.

33 / 33

Help

Keyboard shortcuts

↑, ←, Pg Up, k

Go to previous slide

↓, →, Pg Dn, Space, j

Go to next slide

Home

Go to first slide

End

Go to last slide

Number + Return

Go to specific slide

b / m / f

Toggle blackout / mirrored / fullscreen mode

Clone slideshow

Toggle presenter mode

Restart the presentation timer

?, h

Toggle this help

Prudential fiscal stimulus

Alfred Duncan

University of Kent

Charles Nolan

University of Glasgow

The conventional view (the Greenspan put)

The theorem (Arnott-Greenwald-Stiglitz)

Our contribution

Intuition behind our result

Intuition behind our result

Intuition behind our result

Intuition behind our result

Intuition behind our result

Related literature

The macroprudential externality

The macroprudential externality

Three ingredients

The entrepreneur

The entrepreneur's intratemporal problem

The entrepreneur's intertemporal problem

The household's problem

Factor markets

Factor markets

Factor markets

The competitive allocation of aggregate risk

Optimal macroprudential policy

The macroprudential externality

Optimal wage subsidy policy

Optimal wage subsidy policy - example with log utility

Optimal wage subsidy policy - example with log utility

How the intervention works

Quantitative exercise

Exercise

Wage subsidy simple rule

Expected welfare effects of wage subsidy simple rules

Persistence of TFP shocks

Extensions

Commitment vs discretion

Sticky prices

Summary

The conventional view (the Greenspan put)

Help